The process of Bypass Transition

The growth of the boundary layer

The bypass transition process can be deciphered by undertaking a study of the three main regions concerned with bypass transition [2]:

1) The buffeted laminar boundary layer

2) Region of intermittent turbulent spot formation

3) Fully turbulent boundary layer

The buffeted laminar boundary layer has most of the characteristics of a normal laminar boundary layer. In this region, the skin friction increases only slightly and these perturbations do not affect the stability of the boundary layer. There is shear amplification in this region which leads to the formation of elongated streaks in streamwise direction. As the boundary progresses, these perturbations cause an increase in the instability of the boundary layer leading to the formation of small spots which are turbulent. These spots are termed as turbulent spots and are best explained by Emmons [1]. The spots, initially miniscule in size, tend to grow longitudinally and spread laterally. This region wherein the turbulent spot formation is evident is known as the region of intermittent turbulent spot formation. The turbulent spots ultimately become large and fully turbulent and lead to a fully turbulent boundary layer.

A very important issue in bypass transition is the receptivity of the boundary to external perturbations. Studies conducted by Reshtko [3] and Jacobs & Durbin [2] show that these perturbations enter the boundary layer as very low frequency, low amplitude perturbations. However, these perturbations get amplified and elongated in the streamwise direction by shear to form streamwise streaks. Usually, these perturbations enter the boundary layer in a region where ‘backward jets’ are formed [4]. Backward jets are stream wise streaks that have a negative streamwise velocity. A medium to high FST level is one of the primary causes of these perturbations.

Near wall streaks and its influence on Bypass Transition

DNS simulations by Andersson et al. [5] show that most potential for transient energy amplification is in streamwise aligned vortices. Previously conducted experimental work shows that the receptivity of boundary layer to turbulence depends greatly on the initial conditions and the background noise that forces perturbations into the boundary layer [6] [7]. These experiments reveal that in bypass transition, transition to turbulence can take place at Reynolds numbers well below the critical Reynolds number as proposed by the Linear Stability Theory. When an infinitesimal disturbance is introduced in the boundary layer it has been proven that streamwise velocity component can increase linearly with time and produce alternating low and high velocity streaks. This evolution that the disturbance creates causes a lift-up effect that enables vortices formed (due to the perturbation) in the stream wise direction to advect the mean velocity gradient away from the wall, causing what we term as span wise irregularities or span wise streaks. If the amplitude of these streaks grows to a very large value, there is a tendency for the flow to break down and transition can take place earlier than the value predicted by the linear stability theory. In general, bypass transition is governed by two main instabilities due to streaks viz. the primary instability-the formation of streaks and the secondary instability-enhancement of instabilities in the already generated streamwise streaks. These secondary instabilities depend largely on the infusion of a disturbance in the boundary layer or on the wall surface. A classic example of such a disturbance is the presence of an array of roughness elements on the wall of plate. Experiments conducted by Bakchinov et al. show that the presence of the roughness elements result in the formation of streaks which then get amplified resulting in the instability of the laminar boundary layer to induce transition [8]. Simulations have shown that the most dominant secondary instabilities are sinusoidal in nature [5]. The critical amplitude at which the velocity streaks tend to become unstable is about 26% of the free stream velocity. At 30% streak amplitude it has been shown that breakdown of velocity streaks takes place quite rapidly and results in transition [9]. Experimental investigations by Asai et al. [10] show that a single low speed streak can be develop two different modes of instabilities. One is the symmetric mode of instability which leads to the formation of hairpin shaped vortices which result in the formation of counter rotating streamwise, and the other is the anti-symmetric mode which is sinusoidal and causes meandering of the streak. The anti-symmetric mode has an exponential growth in the boundary layer as compared to the symmetric mode of streak growth. These vortices play a very important role in the breakdown of the laminar boundary layer. According to Asai et al. “streak instability can operate as a dominant mechanism for generating and sustaining coherent structures such as high-speed and low-speed streaks, quasi-streamwise vortices and hairpin vortices”. Experimental data has also suggested that these near wall streamwise streaks play an important role in generating turbulent energy [11]. As explained earlier, the streamwise streak consists of a primary and a secondary instability. Wall turbulence generation usually occurs when high frequency secondary instabilities form hairpin vortices. These hairpin vortices are key elements that trigger the occurrence of a turbulence structure.

Existing methods for Bypass transition study

Several researchers have undertaken comprehensive research to understand the phenomenon of bypass transition. However, the methods adopted by these researches vary from experimental methods to numerical methods like DNS. Some of these methods have been highlighted below and the findings of their work have been highlighted.

Experimental methods

Klingmann [12] made use of a point-like disturbance to develop a streak structure in the boundary layer. It was found that the energy of the streak structure increased linearly and depending on the strength of the disturbance it either decayed or gave rise to a turbulent spot. A critical observation from this experiment is the fact that when turbulent spots occur it is usually due to a secondary instability as explained in 2.2. The stability of streamwise streaks and their effects on bypass transition were studied in detail by Eloffson et al. [13]. The above mentioned authors conducted a study to observe the growth of these streaks and the characteristics that lead to the breakdown of these streaks. The experiments were conducted with the use of hot-wire anemometry with an automatic traversing system that could move in all 3 axis. For the flow visualisation, smoke generators were used and a smoke sheet was introduced into the test section using a transversely oriented slit at the top of the test section. To enable the generation of streaky structures, disturbance were induced using two aluminium plugs of 90 mm diameter were used. The first plug was placed upstream to generate the primary disturbance through a series of five slots through suction and the second plug located downstream generated a time periodic secondary disturbance. The detailed experimental setup is shown in Figure 1. Experimental setup indicating plug positions Due to the presence of suction, high momentum fluid was drawn towards the wall resulting in the formation of alternate regions of high and low velocity. Figure 2 shows the contour plot for the velocity development in the x-z plane at . It can be seen from the figure that upstream primary disturbance results in the formation of streak like structures which have a high velocity as compared to its neighbouring regions.

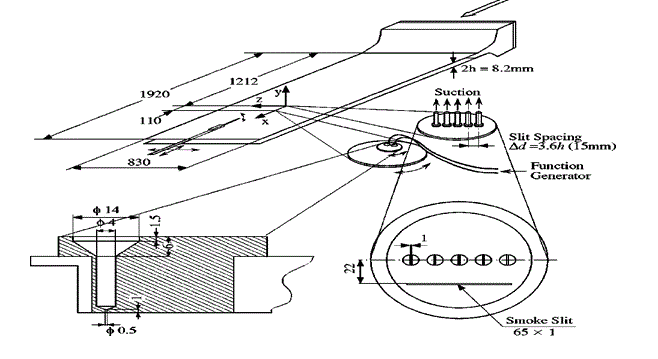
Figure 1. Experimental setup indicating plug positions

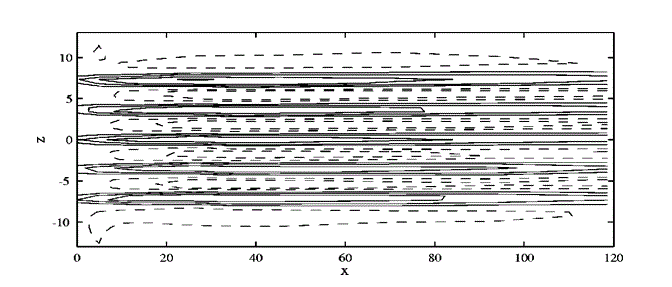
Figure 2. Development of streak like structures

As the streaks progress the plug downstream induces a periodic disturbance in the boundary layer. It was observed that these periodic disturbances result in the formation of two different instability modes viz. the sinuous and the symmetric instability mode. The sinuous mode of instability was found to be the dominant form of instability as found through the hot wire traces. The breakdown of the streaks into turbulence is shown by the contour plot in Figure 3 . This correlates well with the discussion in Near wall streaks and its influence on Bypass Transition that secondary instabilities are primarily responsible for the breakdown of streak like structure which causes transition.

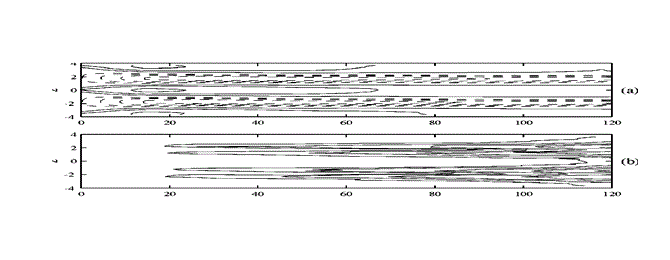
Figure 3. a) Development of streaky structure b) Consequent breakdown of streaky structure

It was observed through this experiment that the streak amplitude grows and if this amplitude exceeds a certain threshold, secondary instability in the form of sinusoidal waves bypass transition takes place.

Another experiment of important consequence to this paper is a study of wake-induced bypass transition [14]. The authors in this case subjected a flat plate to wakes in a wind tunnel. The experimental setup in this case consisted of a wind tunnel with a FSTI of 0.5% which could be increased to 1.5% using turbulent grid generators. Periodic wakes were produced with the use of a disc with two cylindrical bars mounted on a spokenwheel generator. The unsteady velocity field in this case was measured using hot wire anemometry with an automatic traversing system. The flat plate was a 1m plate with an elliptic leading edge of 1:5 AR. Readings were taken at 21 different locations on the plate to study the effects of these wakes on bypass transition. Figure 4 shows the test experimental facility in detail.

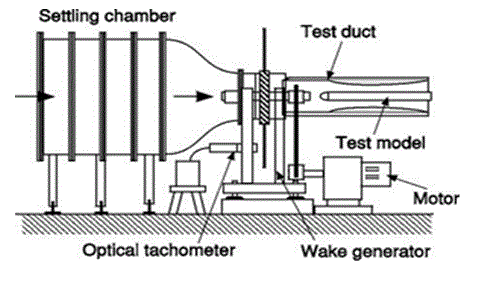
Figure 4. Experimental test setup

The raw velocity data collected from the experiment at a FSTI showed an abrupt fluctuation in the velocity at around as clearly exemplified in Figure 5 .

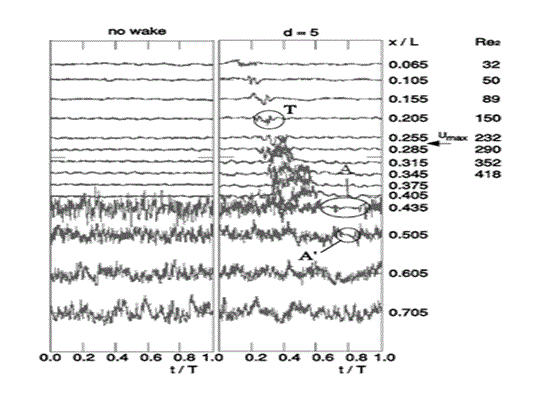
Figure 5. Raw velocity date indicating velocity fluctuations

There is clear evidence from the raw velocity signals that the induced wake led to an increase in velocity fluctuation which corroborates the theory that an external disturbance is essential to trigger bypass transition. The time averaged intermittency for Test case1 (no wake) and Test case 2(induced wake) show that the wake induces a wedge shaped trace, which implies that the wake passage induced transition through production of turbulent spots. The graph in Figure 6 clearly indicates the region of wake induced transition.

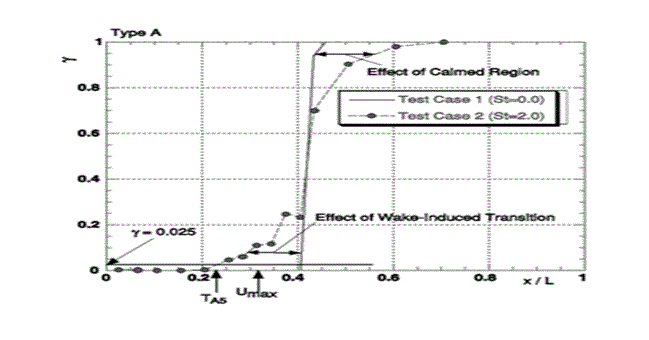
Figure 6. Intermittency function indicating wake induced transition trace

The effects of free-stream turbulence on the bypass transition were also studied. It was found that with an increase in FSTI levels from 0.5% to 1.5% the transition tends to arrive much earlier and there is a further promotion of bypass transition with enhanced intensity. The authors however do comment and are of the opinion that although their data correlates with existing data, the physics of bypass transition through wake induction remains very unclear within the boundary layer and is possibly very similar to the existing promoted theories of bypass transition mechanisms.

Bibliography

Andersson, P., et al. “On the breakdown of boundary layer streaks .” J. Fluid Mech. 428 (2001): 29-60.

Asai, M., M. Minagawa and M. Nishioka. “The instability and breakdown of a near-wall low-speed streak.” J. Fluid Mech. 455 (2002): 289-314.

Bakchinov, A.A., G.R.l Klingmann,B.G.B. Grek and V.V. Kozlov. “Transition experiments in a boundary layer with embedded streamwise vortices.” Phys. Fluids 7 (1995): 820.

Berlin, S., A. Lundbladh and D.S. Henningson. “Spatial simulations of oblique transition in a boundary layer.” Phys. Fluids 6 (1994): 1949.

Elofsson, P.A., M. Kawakami and P.H. Alfredsson. “Experiments on the stability of streamwise streaks in plane Poiseuille flow.” Phys. Fluids 11 (1999): 915.

Emmons, H. “The Laminar-Turbulent Transition in a Boundary Layer-Part I.” Journal of Aeronautical Sciences 18.7 (1951): 490-498.

Jacobs, R.G. and P.A. Durbin. “Simulations of bypass transition.” Journal of Fluid Mechanics 428 (2001): 185-212.

Kline, S.J., et al. “The structure of turbulent boundary layers.” J. Fluid Mech. 30 (1997): 741-773.

Klingmann, B.G.B. “On transition due to three-dimensional disturbances in plane Poiseuille flow.” J. Fluid Mech. 240 (1992): 167.

Koyabu, E., K. Funazaki and M. Kimura. “Experimental studies of wake-induced bypass transition of flat-plate boundary layers under favorable and adverse pressure gradients.” JSME Int. Jou. 48.3 (2005): 579-588.

Morkovin, M.V. and E. Reshotko. “Dialogue on process and issues in stability and transition research.” Arnal, D. and R. Michel. Laminar-Turbulent Transition. Springer, 1990.

Reshotko, E. “Boundary layer instability, transition and control.” AIAA (1994).

Reshotko, E.A. “Boundary layer stability and transition.” Ann.Rev.Fluid Mech 8 (1976): 311-349.

Wu, X., et al. “Simulation of boundary layer transition induced by periodically passing wakes.” J. Fluid Mech. 398 (1999): 193-218.